h-net: hqc1103

Topological data

Orbifold symbol	*22222
Transitivity (vertex, edge, ring)	(3,5,2)
Vertex degrees	{8,3,4}
2D vertex symbol	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}
Delaney-Dress Symbol	<1103.2:9:1 3 5 6 8 9,2 3 6 7 9,1 4 5 6 7 8 9:3 6,8 3 4>
Dual net	hqc987

Derived s-nets

s-nets with faithful topology

20 records listed.

Image	s-net name	Other names	Space group	Space group number	Symmetry class	Vertex degree(s)	Vertices per primitive unit cell	Transitivity (Vertex, Edge)
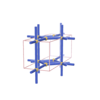
	sqc226		Pmmm	47	orthorhombic	{3,8,4}	4	(3,5)
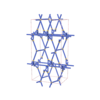
	sqc2613		Fmmm	69	orthorhombic	{4,3,8}	8	(3,5)
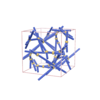
	sqc8418		I4122	98	tetragonal	{8,3,4}	16	(3,6)
	sqc8500		Fddd	70	orthorhombic	{8,3,4}	16	(3,6)
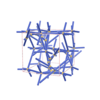
	sqc8501		I4122	98	tetragonal	{8,3,4}	16	(3,6)
	sqc8631		Fddd	70	orthorhombic	{8,3,4}	16	(3,6)
	sqc8646		I4122	98	tetragonal	{8,3,4}	16	(3,6)
	sqc8653		I4122	98	tetragonal	{8,3,4}	16	(3,6)
	sqc8821		Fddd	70	orthorhombic	{8,3,4}	16	(3,6)
	sqc8822		I4122	98	tetragonal	{8,3,4}	16	(3,6)
	sqc8823		Fddd	70	orthorhombic	{8,3,4}	16	(3,6)
	sqc8851		Fddd	70	orthorhombic	{8,3,4}	16	(3,6)
	sqc2297		P4222	93	tetragonal	{3,4,8}	8	(3,5)
	sqc2326		P4222	93	tetragonal	{3,4,8}	8	(3,5)
	sqc2372		P42/mmc	131	tetragonal	{3,4,8}	8	(3,5)
	sqc2424		P4222	93	tetragonal	{3,4,8}	8	(3,5)
	sqc2502		P4222	93	tetragonal	{4,3,8}	8	(3,5)
	sqc2563		Cmma	67	orthorhombic	{3,4,8}	8	(3,5)
	sqc2614		Cmma	67	orthorhombic	{8,3,4}	8	(3,5)
	sqc2872		Cmma	67	orthorhombic	{4,3,8}	8	(3,5)

s-nets with edge collapse

show / hide

Derived U-tilings

10 records listed.

Image	U-tiling name	PGD Subgroup	Transitivity (Vert,Edge,Face)	Vertex Degree	Vertex Symbol	P net	G net	D net
	UQC3865	*22222a	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc2134	sqc8501	sqc2326
	UQC3866	*22222a	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc8272	sqc8822	sqc2297
	UQC3867	*22222a	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc8340	sqc8646	sqc2372
	UQC3868	*22222a	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc8342	sqc8653	sqc2424
	UQC3869	*22222b	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc226	sqc8851	sqc2614
	UQC3870	*22222a	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc8267	sqc8418	sqc2502
	UQC3871	*22222b	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc2255	sqc8631	sqc226
	UQC3872	*22222b	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc2251	sqc8500	sqc2563
	UQC3873	*22222b	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc2613	sqc8821	sqc226
	UQC3874	*22222b	(3,5,2)	{8,3,4}	{3.6.6.3.3.6.6.3}{3.6.6}{6.6.6.6}	sqc2253	sqc8823	sqc2872

Symmetry-lowered hyperbolic tilings

show / hide