h-net: hqc2243

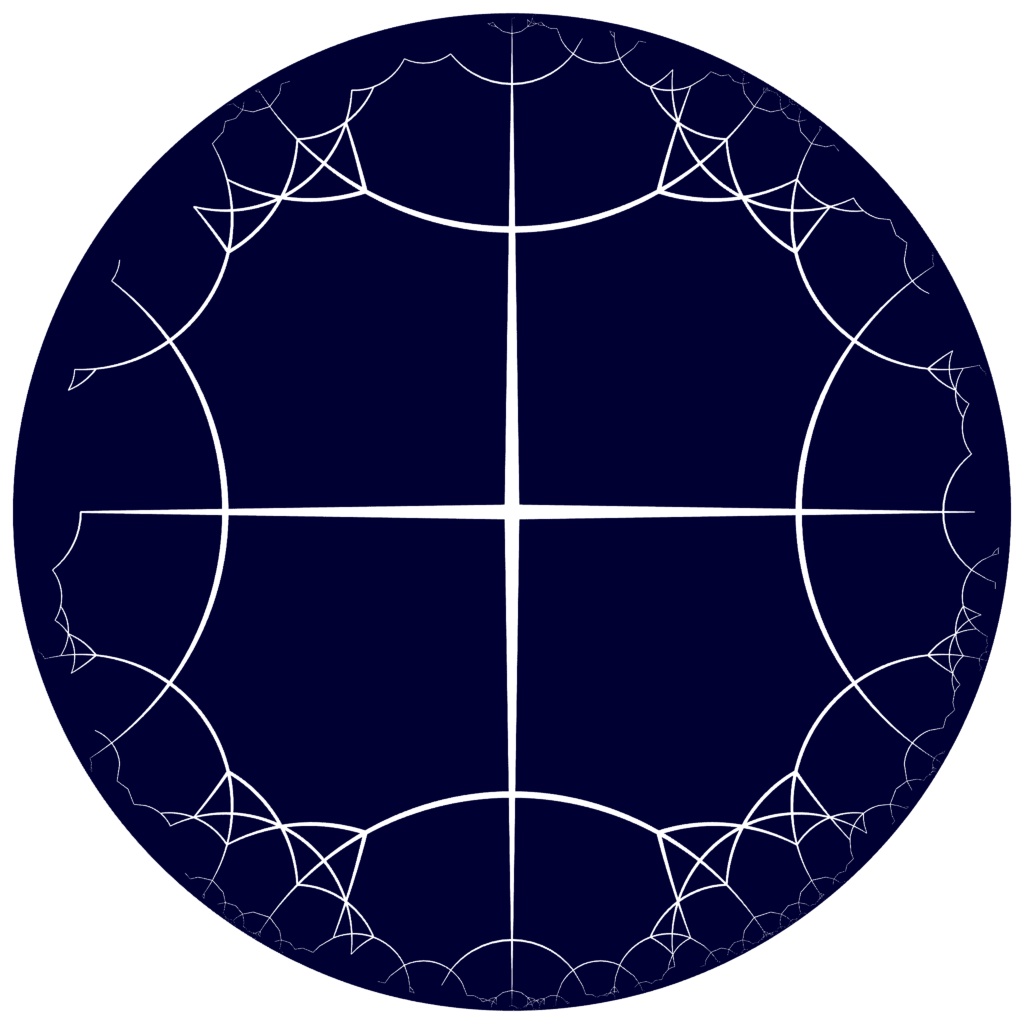
Topological data

Orbifold symbol	*22222
Transitivity (vertex, edge, ring)	(5,6,2)
Vertex degrees	{4,4,4,6,4}
2D vertex symbol	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3.3.7.3.3}{3.3.3.3}
Delaney-Dress Symbol	<2243.2:13:1 3 5 7 9 11 13,2 4 6 7 10 13 12,1 2 3 4 5 8 9 10 11 12 13:7 3,4 4 4 6 4>
Dual net	hqc2174

Derived s-nets

s-nets with faithful topology

23 records listed.

Image	s-net name	Other names	Space group	Space group number	Symmetry class	Vertex degree(s)	Vertices per primitive unit cell	Transitivity (Vertex, Edge)
	sqc5894		Fmmm	69	orthorhombic	{4,6,4,4,4}	12	(5,6)
	sqc5997		Fmmm	69	orthorhombic	{4,6,4,4,4}	12	(5,6)
	sqc11492		P4/mmm	123	tetragonal	{4,4,4,6,4}	24	(5,6)
	sqc11391		I4122	98	tetragonal	{4,4,4,6,4}	24	(5,7)
	sqc11392		Fddd	70	orthorhombic	{4,4,4,6,4}	24	(5,7)
	sqc11401		I4122	98	tetragonal	{4,4,4,6,4}	24	(5,7)
	sqc11407		Fddd	70	orthorhombic	{4,4,4,6,4}	24	(5,7)
	sqc11408		Fddd	70	orthorhombic	{4,4,4,6,4}	24	(5,7)
	sqc11423		I4122	98	tetragonal	{4,4,4,6,4}	24	(5,7)
	sqc11493		I4122	98	tetragonal	{4,4,4,6,4}	24	(5,7)
	sqc11494		Fddd	70	orthorhombic	{4,4,4,6,4}	24	(5,7)
	sqc11499		I4122	98	tetragonal	{4,4,4,6,4}	24	(5,7)
	sqc11507		Fddd	70	orthorhombic	{4,4,4,6,4}	24	(5,7)
	sqc1048		Pmmm	47	orthorhombic	{4,6,4,4,4}	6	(5,6)
	sqc5625		P4222	93	tetragonal	{6,4,4,4,4}	12	(5,6)
	sqc5668		P4222	93	tetragonal	{4,4,6,4,4}	12	(5,6)
	sqc5767		P4222	93	tetragonal	{4,4,4,6,4}	12	(5,6)
	sqc5925		Cmma	67	orthorhombic	{4,4,4,6,4}	12	(5,6)
	sqc6000		Cmma	67	orthorhombic	{4,4,6,4,4}	12	(5,6)
	sqc6009		Cmma	67	orthorhombic	{4,4,4,6,4}	12	(5,6)
	sqc6010		P42/mmc	131	tetragonal	{4,4,4,6,4}	12	(5,6)
	sqc6011		P4222	93	tetragonal	{4,4,4,6,4}	12	(5,6)

s-nets with edge collapse

show / hide

Derived U-tilings

10 records listed.

Image	U-tiling name	PGD Subgroup	Transitivity (Vert,Edge,Face)	Vertex Degree	Vertex Symbol	P net	G net	D net
	UQC5664	*22222a	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	No s‑net	sqc11493	sqc5625
	UQC5665	*22222a	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc11283	sqc11391	sqc5668
	UQC5666	*22222b	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	No s‑net	sqc11392	sqc5925
	UQC5667	*22222b	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc5620	sqc11407	sqc6000
	UQC5668	*22222b	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc5997	sqc11494	sqc1048
	UQC5669	*22222a	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	No s‑net	sqc11401	sqc5767
	UQC5670	*22222b	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc1048	sqc11507	sqc6009
	UQC5671	*22222b	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc5894	sqc11408	sqc1048
	UQC5672	*22222a	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc11282	sqc11423	sqc6011
	UQC5673	*22222a	(5,6,2)	{4,4,4,6,4}	{7.7.7.7}{7.7.7.7}{7.3.3.7}{7.3....	sqc11492	sqc11499	sqc6010

Symmetry-lowered hyperbolic tilings

show / hide