The Primitive (P) surface

This is one of three closely-related triply periodic minimal surfaces of cubic symmetry and genus three (the other examples are the D and G surfaces). The P surface was first described and parametrised by the celebrated 19th century analyst, Hermann Amandus Schwarz in 1865, though his illustrious predecessors, Riemann and Weierstrass also contributed to the problem (an account can be found in Nitsche ). For some reason, physicists have named soft materials adopting this structure “plumbers nightmare phases”. To us (admittedly apprentice plumbers) all other structures based on triply periodic minimal surfaces appear far more nightmarish than the P!

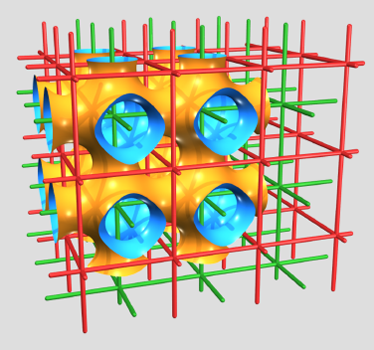
P surface and labyrinth (image courtesy Gerd Schröder-Turk)

The P surface is balanced , with a pair of identical sub-volumes on either side of the surface. The labyrinth graph of each sub-volume is the 6-coordinated jungle gym pattern, the graph connecting nearest vertices of the primitive cubic lattice (called pcu in the Reticular Chemistry Structure Database.

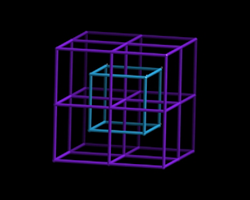
Interwoven cubic nets

The 3D euclidean symmetry–pair for this surface is Im-3m / Pm-3m, where the former space group (that of the non-oriented surface) refers to symmetries that include operations which exchange sides of the surface, and the latter (oriented symmetry) excludes those operations (and is therefore the symmetry of a single sub-volume on one side of the surface). Note that lower-symmetry triply periodic minimal surface variants of the P surface exist, including tetragonal (tP), rhombohedral (rPD), and orthorhombic (oPa, oPb) examples.

The 2D hyperbolic symmetry of this surface has Conway orbifold symbol *246. A single asymmetric surface patch of the P surface is bounded by two mirror planes meeting at a dihedral angle of π/4, and a straight line (defining a two-fold axis of symmetry that distinguishes Im-3m from Pm-3m). A pair of patches, sharing a common straight line, is therefore bounded by four mirror planes with dihedral angles π/2, π/2, π/2, and π/3. The corresponding hyperbolic orbifold is *2223. This surface patch lies inside a quadrirectangular tetrahedron — the asymmetric volume of the Pm-3m space group listed in the International Tables for Crystallography. See Brakke's site for more images.

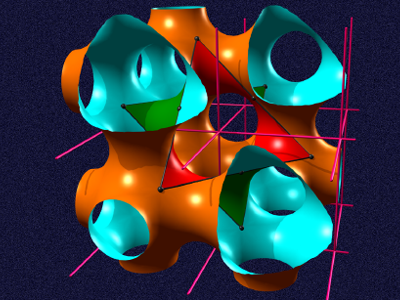
(image courtesy Gerd Schröder-Turk)

Alternatively, the P surface can be built up from a surface patch bounded completely by two-fold axes (linear asymptotes), with rotations of π/2 around each straight edge. A nonplanar quadrilateral is the smallest linear boundary for a patch of the P surface containing no interior two-fold axes. Six such elements are bounded by the Petrie polygon of the platonic octahedron, and the edges form the net sqc5432.

Return to the EPINET mathematical background page.