Two-dimensional Orbifolds

A two-dimensional orbifold encodes the symmetric properties of an infinitely repeating 2D pattern with the topological features of a compact, connected surface. The concept has its roots in the theory of discrete groups, but the name was coined by Bill Thurston and his students by combining the terms orbit and manifold , since each point of an orbifold represents the entire orbit of a point under the group of symmetry operations of the pattern. Alternatively, you can think of the orbifold surface as “rolling around” on one of the three 2D geometries - the sphere, S², euclidean, E², or hyperbolic, H², planes - decorating it as it goes. Technically, the orbifold is the quotient space of the pattern by its symmetry group, and the pattern is the universal cover of the orbifold.

Some Euclidean examples

*x or cm	333 or p3

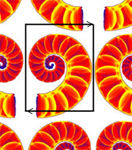
The euclidean tiling pattern labelled *x above has reflection symmetries, translations, and glides but no rotational symmetry. The asymmetric unit is the rectangle cut out as shown below the tiling. The vertical sides are mirror lines of the reflection symmetries. The glide symmetry matches the bottom edge to the top edge but in the opposite direction. This region glues up to a möbius band orbifold (also shown above) with its single edge as a mirror boundary. The Conway orbifold symbol is *x and the plane crystallographic symbol is cm .

The tiling pattern labelled 333 has only rotational symmetries. An asymmetric unit is the quadrilateral outlined below the tiling, and this region glues up into a pillow with three corners.

Technical details

Two-dimensional symmetries are rotations about a point, reflections in mirror lines (that may intersect or be parallel), translations, and glide reflections. In the orbifold a reflection produces a boundary component, a rotation induces a cone point, while translations and glides that do not arise from other symmetries of the pattern are encoded by global topological features such as rings, handles, and crosscaps. These relationships are described in more detail below.

A cone point is just what its name suggests. Rolling a cone about its apex will generate a pattern with rotational symmetry at that point. For the pattern to repeat exactly, the angle swept out by the cone must be an integer subdivision of 360°. An orbifold with just three cone points looks like a samosa. If there are only two cones each cone must be of the same order, as they represent opposite poles of a spherical pattern. There are no compact orbifolds containing a single cone point.

Now suppose the orbifold has a puncture, and therefore a boundary edge. If you are rolling the surface out over its universal cover, or if you are travelling along it and you hit an edge, you can imagine flipping or crossing over to the other side of the orbifold, where the pattern is locally reversed. Boundaries therefore encode mirror reflections. A puncture boundary must be a closed loop, which may contain sharp corners. A kaleidoscopic orbifold is one that has a single mirror boundary and no other topological features. A corner in the orbifold is formed by two mirror lines meeting at an angle less than 180°. As with the cone points, for the pattern to unwrap and meet itself exactly, only those angles which are integer subdivisions of 180° are allowed.

Cone and corner points are local features of an orbifold and they are inherintly non-manifold (as there is no disc-like neighbourhood isomorphic to E²). This means that an orbifold is not a true manifold.

A translational symmetry may be induced by combinations of reflections or rotations, but if this is not the case, a translation will be encoded by a non-trivial loop in the orbifold, as found on a torus (also called a handle), or a ribbon with its ends glued together in an orientable way. It is simple enough to imagine a such a glued ribbon rolling across the euclidean plane and mapping out a translationally periodic pattern (its boundaries will represent mirror lines).

A glide reflection is the (irreducible) composition of a translation with a reflection, and is encoded by a non-orientable surface feature such as a möbius strip or crosscap. One traversal of the loop along the center of a möbius strip brings you to the opposite side of the ribbon; a second traversal is needed to come back to the starting point on the correct side. In a group theoretic sense, this tells us that applying a glide symmetry twice gives a translation.

The central result of 2-manifold topology is that every closed, compact, two-dimensional manifold is topologicially equivalent to either the sphere, a sphere with n handles attached, or a sphere with n crosscaps. A 2D orbifold is a 2-manifold with a finite number of punctures, corner, and cone points, so a compact description of these features can encode any discrete symmetry group of the sphere, euclidean, and hyperbolic planes.

A simple notation for orbifolds is due to John Conway (and derived from Macbeath's non-euclidean crystallographic group signature). From it we can reconstruct the orbifold surface topology, and all its associated symmetry features. The notation is unique up to certain rearrangements of elements. The global topological features are the handles, crosscaps, and boundaries, and are denoted by the symbols 'o', 'x', and '*'. Note that handles and crosscaps never coexist, as a handle transforms to two crosscaps in the presence of another crosscap (ox = xxx). Thus our symbol will either be prefixed by 'o's or suffixed by 'x's, one for each topological element. The non-manifold features (cone and corner points) are represented by the integer order of the symmetry operation at that point, or equivalently, the integer fractions of 360° and 180° respectively.

As there is no inherent ordering to non-boundary points of a manifold, the cone points may be listed in any order. Thus, the orbifold symbol 457 is equivalent to 754 or even 475 - in fact any permutation is valid. We typically use lexical ordering to make a nice canonical form, but this is not intrinsic to the notation. Corner points, however, have a distinct ordering based on their sequence around a boundary component. A mirror string is given by a single '*', representing the boundary component, followed by a list of its corner points (if any). Because order matters, *4567 is different to *4765. However, because there is no intrinsic start or end to a mirror boundary, any cyclic permutation is equivalent, i.e. *5674 and *6745 represent the same orbifold as *4567. Also, because a punctured surface has no intrinsic inside or outside, you can reverse the order of the corner points: i.e. *4567 is equivalent to *7654. Entire mirror strings correspond to distinct punctures, and like cone points, there is no way to intrinsically order these features. Therefore the order of the mirror strings does not matter.

The complete lexical specification of an orbifold symbol is therefore:
[some number of o's][cone points in any order][mirror strings in any order][some number of x's]
ooo... c1c2c3... *m1m2... *m4m5... ...xxx

A 2D orbifold has an associated scalar value called the curvature which can be computed from its symbolic elements. The formula is:

K = 2 - 2*[no. of 'o's] - [no. of '*'s] - [no. of 'x's]
- [sum over cone points of (ci-1)/ci]
- [sum over corner points of (mi-1)/(2*mi)]

The curvature value determines which of the three 2D geometries the symmetry pattern must belong to (S2, E2, or H2). If K > 0, the symmetry is spherical, K=0 implies a euclidean pattern, and K < 0 only for patterns with hyperbolic symmetry. In the case of spherical and hyperbolic geometry, the curvature essentially measures the area of a single image of the orbifold. Euclidean space is unique in that shapes may be scaled arbitrarily whilst preserving angles, so a Euclidean orbifold has no associated area. This leads to a simple technique for enumerating the 17 wallpaper groups, as demonstrated in Conway's papers (referenced in the bibliography).

Return to the EPINET mathematical background page.