Fddd

Number	70
Symmetry Class	orthorhombic
Chiral	N

s-nets

816 records listed.

Image	s-net name	Other names	Space group	Space group number	Symmetry class	Vertex degree(s)	Vertices per primitive unit cell	Transitivity (Vertex, Edge)
	sqc7469		Fddd	70	orthorhombic	{12,4}	8	(2,5)
	sqc7470		Fddd	70	orthorhombic	{12,4}	8	(2,5)
	sqc7471		Fddd	70	orthorhombic	{6,10}	8	(2,5)
	sqc7472		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7473		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7474		Fddd	70	orthorhombic	{4,8}	12	(2,5)
	sqc7475		Fddd	70	orthorhombic	{12,4}	8	(2,5)
	sqc7476		Fddd	70	orthorhombic	{6,10}	8	(2,5)
	sqc7478		Fddd	70	orthorhombic	{12,4}	8	(2,5)
	sqc7481		Fddd	70	orthorhombic	{8,4}	12	(2,5)
	sqc7482		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7483		Fddd	70	orthorhombic	{6,10}	8	(2,5)
	sqc7485		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7493		Fddd	70	orthorhombic	{4,8,4}	12	(3,5)
	sqc7514		Fddd	70	orthorhombic	{6,10}	8	(2,5)
	sqc7516		Fddd	70	orthorhombic	{12,4}	8	(2,5)
	sqc7526		Fddd	70	orthorhombic	{4,8}	12	(2,5)
	sqc7527		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7528		Fddd	70	orthorhombic	{4,8}	12	(2,5)
	sqc7529		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7530		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7531		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7547		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7552		Fddd	70	orthorhombic	{4,8,4}	12	(3,5)
	sqc7567		Fddd	70	orthorhombic	{3,10}	12	(2,5)
	sqc7584		Fddd	70	orthorhombic	{6,10}	8	(2,5)
	sqc7589		Fddd	70	orthorhombic	{4,6}	12	(2,5)
	sqc7621		Fddd	70	orthorhombic	{4,6}	12	(2,5)
	sqc7636		Fddd	70	orthorhombic	{6,4}	12	(2,5)
	sqc7638		Fddd	70	orthorhombic	{6,4}	12	(2,5)
	sqc7646		Fddd	70	orthorhombic	{4,3,6}	16	(3,5)
	sqc7647		Fddd	70	orthorhombic	{6,4}	12	(2,5)
	sqc7649		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7651		Fddd	70	orthorhombic	{6,4}	12	(2,5)
	sqc7657		Fddd	70	orthorhombic	{6,4}	12	(2,5)
	sqc7660		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7662		Fddd	70	orthorhombic	{6,4}	12	(2,5)
	sqc7663		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7665		Fddd	70	orthorhombic	{6,3,4}	16	(3,5)
	sqc7666		Fddd	70	orthorhombic	{4,3,6}	16	(3,5)
	sqc7667		Fddd	70	orthorhombic	{4,3,6}	16	(3,5)
	sqc7673		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7677		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7678		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7679		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7680		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7682		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7683		Fddd	70	orthorhombic	{6,5}	12	(2,5)
	sqc7686		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7688		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7689		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7690		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7691		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7697		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7698		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7700		Fddd	70	orthorhombic	{3,5}	16	(2,5)
	sqc7704		Fddd	70	orthorhombic	{8,4,4}	12	(3,5)
	sqc7708		Fddd	70	orthorhombic	{6,6,4}	12	(3,5)
	sqc7709		Fddd	70	orthorhombic	{3,6,4}	16	(3,5)
	sqc7711		Fddd	70	orthorhombic	{4,6,3}	16	(3,5)
	sqc7714		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7735		Fddd	70	orthorhombic	{4,8}	12	(2,5)
	sqc7736		Fddd	70	orthorhombic	{4,6}	12	(2,5)
	sqc7737		Fddd	70	orthorhombic	{4,6}	12	(2,5)
	sqc7738		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7739		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7745		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7749		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7752		Fddd	70	orthorhombic	{4,4}	16	(2,5)
	sqc7754		Fddd	70	orthorhombic	{4,4}	16	(2,5)
	sqc7755		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7757		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
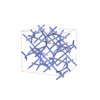
	sqc7760		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7761		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7762		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7763		Fddd	70	orthorhombic	{8,4,4}	12	(3,5)
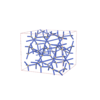
	sqc7764		Fddd	70	orthorhombic	{8,4,4}	12	(3,5)
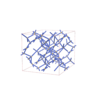
	sqc7765		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7767		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7769		Fddd	70	orthorhombic	{6,6,4}	12	(3,5)
	sqc7774		Fddd	70	orthorhombic	{6,6,4}	12	(3,5)
	sqc7776		Fddd	70	orthorhombic	{4,6,3}	16	(3,5)
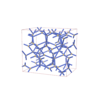
	sqc7777		Fddd	70	orthorhombic	{3,6,4}	16	(3,5)
	sqc7778		Fddd	70	orthorhombic	{3,6,4}	16	(3,5)
	sqc7779		Fddd	70	orthorhombic	{4,6,3}	16	(3,5)
	sqc7784		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7785		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7786		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7787		Fddd	70	orthorhombic	{4,4,4}	16	(3,5)
	sqc7792		Fddd	70	orthorhombic	{3,3,4}	20	(3,5)
	sqc7793		Fddd	70	orthorhombic	{6,3,4}	16	(3,5)
	sqc7794		Fddd	70	orthorhombic	{4,3,6}	16	(3,5)
	sqc7795		Fddd	70	orthorhombic	{6,3,4}	16	(3,5)
	sqc7796		Fddd	70	orthorhombic	{6,3,4}	16	(3,5)
	sqc7798		Fddd	70	orthorhombic	{4,3,3}	20	(3,5)
	sqc7803		Fddd	70	orthorhombic	{3,3,4}	20	(3,5)
	sqc7805		Fddd	70	orthorhombic	{3,3,4}	20	(3,5)
	sqc7806		Fddd	70	orthorhombic	{3,3,4}	20	(3,5)
	sqc7807		Fddd	70	orthorhombic	{4,3,3}	20	(3,5)
	sqc7808		Fddd	70	orthorhombic	{4,3,3}	20	(3,5)