Fddd

Number	70
Symmetry Class	orthorhombic
Chiral	N

s-nets

816 records listed.

Image	s-net name	Other names	Space group	Space group number	Symmetry class	Vertex degree(s)	Vertices per primitive unit cell	Transitivity (Vertex, Edge)
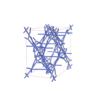
	sqc10268		Fddd	70	orthorhombic	{8,3,4,4}	20	(4,6)
	sqc10272		Fddd	70	orthorhombic	{8,3,4,4}	20	(4,6)
	sqc10273		Fddd	70	orthorhombic	{8,3,4,4}	20	(4,6)
	sqc10280		Fddd	70	orthorhombic	{5,6}	16	(2,7)
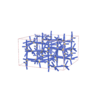
	sqc10281		Fddd	70	orthorhombic	{5,6}	16	(2,7)
	sqc10283		Fddd	70	orthorhombic	{5,6}	16	(2,7)
	sqc10286		Fddd	70	orthorhombic	{6,5}	16	(2,7)
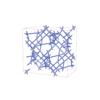
	sqc10290		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10292		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
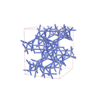
	sqc10294		Fddd	70	orthorhombic	{6,5}	16	(2,7)
	sqc10295		Fddd	70	orthorhombic	{6,5}	16	(2,7)
	sqc10296		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10298		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10304		Fddd	70	orthorhombic	{6,5}	16	(2,7)
	sqc10305		Fddd	70	orthorhombic	{6,5}	16	(2,7)
	sqc10310		Fddd	70	orthorhombic	{5,3,3}	24	(3,6)
	sqc10311		Fddd	70	orthorhombic	{5,3,3}	24	(3,6)
	sqc10315		Fddd	70	orthorhombic	{5,3,3}	24	(3,6)
	sqc10316		Fddd	70	orthorhombic	{5,3,3}	24	(3,6)
	sqc10324		Fddd	70	orthorhombic	{4,8,6,4}	16	(4,6)
	sqc10327		Fddd	70	orthorhombic	{4,4,4,3}	24	(4,7)
	sqc10329		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10331		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10349		Fddd	70	orthorhombic	{4,4,3,4}	24	(4,7)
	sqc10351		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10353		Fddd	70	orthorhombic	{4,8,6,4}	16	(4,6)
	sqc10360		Fddd	70	orthorhombic	{4,8,6,4}	16	(4,6)
	sqc10364		Fddd	70	orthorhombic	{4,4,4,3}	24	(4,7)
	sqc10365		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10366		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10367		Fddd	70	orthorhombic	{4,4,4,3}	24	(4,7)
	sqc10368		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10369		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10370		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10371		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10372		Fddd	70	orthorhombic	{4,4,3,4}	24	(4,7)
	sqc10378		Fddd	70	orthorhombic	{4,4,3,4}	24	(4,7)
	sqc10379		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10380		Fddd	70	orthorhombic	{4,4,6,4}	20	(4,6)
	sqc10381		Fddd	70	orthorhombic	{4,4,6,4}	20	(4,6)
	sqc10382		Fddd	70	orthorhombic	{4,4,6,4}	20	(4,6)
	sqc10390		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10392		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10393		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10394		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10397		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10398		Fddd	70	orthorhombic	{4,4,3,4}	24	(4,7)
	sqc10403		Fddd	70	orthorhombic	{7,4}	16	(2,7)
	sqc10405		Fddd	70	orthorhombic	{8,6,4,4}	16	(4,6)
	sqc10407		Fddd	70	orthorhombic	{3,8}	16	(2,7)
	sqc10408		Fddd	70	orthorhombic	{3,8}	16	(2,7)
	sqc10409		Fddd	70	orthorhombic	{8,3}	16	(2,7)
	sqc10410		Fddd	70	orthorhombic	{3,8}	16	(2,7)
	sqc10411		Fddd	70	orthorhombic	{3,8}	16	(2,7)
	sqc10412		Fddd	70	orthorhombic	{3,8}	16	(2,7)
	sqc10414		Fddd	70	orthorhombic	{7,4}	16	(2,7)
	sqc10415		Fddd	70	orthorhombic	{7,4}	16	(2,7)
	sqc10417		Fddd	70	orthorhombic	{8,6,4,4}	16	(4,6)
	sqc10418		Fddd	70	orthorhombic	{8,6,4,4}	16	(4,6)
	sqc10419		Fddd	70	orthorhombic	{8,6,4,4}	16	(4,6)
	sqc10421		Fddd	70	orthorhombic	{5,6}	16	(2,7)
	sqc10422		Fddd	70	orthorhombic	{5,6}	16	(2,7)
	sqc10427		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10428		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10432		Fddd	70	orthorhombic	{5,3,3}	24	(3,6)
	sqc10444		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10448		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10449		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10450		Fddd	70	orthorhombic	{6,4,4}	20	(3,6)
	sqc10456		Fddd	70	orthorhombic	{8,6,4,4}	16	(4,6)
	sqc10460		Fddd	70	orthorhombic	{8,3,4,4}	20	(4,6)
	sqc10461		Fddd	70	orthorhombic	{8,3,4,4}	20	(4,6)
	sqc10489		Fddd	70	orthorhombic	{4,8,6,4}	16	(4,6)
	sqc10490		Fddd	70	orthorhombic	{4,8,6,4}	16	(4,6)
	sqc10516		Fddd	70	orthorhombic	{4,4,6,4}	20	(4,6)
	sqc10518		Fddd	70	orthorhombic	{4,4,6,4}	20	(4,6)
	sqc10540		Fddd	70	orthorhombic	{4,6,4,4}	20	(4,6)
	sqc10542		Fddd	70	orthorhombic	{4,6,4,4}	20	(4,6)
	sqc10544		Fddd	70	orthorhombic	{4,6,4,4}	20	(4,6)
	sqc10545		Fddd	70	orthorhombic	{4,6,4,4}	20	(4,6)
	sqc10546		Fddd	70	orthorhombic	{4,6,4,4}	20	(4,6)
	sqc10584		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10585		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10596		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10622		Fddd	70	orthorhombic	{3,4,4,4}	24	(4,7)
	sqc10623		Fddd	70	orthorhombic	{4,4,4,3}	24	(4,7)
	sqc10624		Fddd	70	orthorhombic	{4,4,4,3}	24	(4,7)
	sqc10625		Fddd	70	orthorhombic	{4,3,4,4}	24	(4,7)
	sqc10626		Fddd	70	orthorhombic	{4,4,3,4}	24	(4,7)
	sqc10650		Fddd	70	orthorhombic	{3,4,4}	24	(3,6)
	sqc10656		Fddd	70	orthorhombic	{3,4,4}	24	(3,6)
	sqc10657		Fddd	70	orthorhombic	{3,4,4}	24	(3,6)
	sqc10658		Fddd	70	orthorhombic	{3,4,4}	24	(3,6)
	sqc10659		Fddd	70	orthorhombic	{3,4,4}	24	(3,6)
	sqc10712		Fddd	70	orthorhombic	{12,3,3}	20	(3,7)
	sqc10713		Fddd	70	orthorhombic	{12,3,3}	20	(3,7)
	sqc10715		Fddd	70	orthorhombic	{12,3,3}	20	(3,7)
	sqc10736		Fddd	70	orthorhombic	{12,3,3}	20	(3,7)
	sqc10737		Fddd	70	orthorhombic	{12,3,3}	20	(3,7)
	sqc10781		Fddd	70	orthorhombic	{8,4,4}	20	(3,7)